Identical Triangles Card Sort

Identifying identical triangles was a little bit of a challenge for a few of my 7th grade students.   They could correctly identify a property (Angle, Angle, Side), but then would completely disregard the fact that the sides in the two triangles were not corresponding.    I want to revisit the concept with these students as a quick review activity even though our lessons have moved on to other topics.   To do so, I created a card sort.

In the card sort, each card has a pair of triangles.    Students will sort the cards into categories:   “Identical”, “Not Identical”, or “Not Enough Information To Tell”.   If the triangles are identical, they will be required to identify the property shown.   In order to address the fact that students are not looking to see if the sides are corresponding, I will probably require them to use colored dry erase markers to indicate corresponding sides.

indentical-triangle-card-sort

I will pair students who did not master the concept with students who did.   I think I will implement a strategy that I read about on another blog (I don’t remember which one, only that it was a great idea), in which students within the pairing are required to take turns.   The first partner sorts a card into a category.   The second partner then either agrees or disagrees with the sorting.   If he or she disagrees, he or she can move it but must explain why it is being moved.   The second partner then sorts another card and the first partner can agree or disagree.

Save

Angle Card Sets – Exploring Angle Relationships and Solving Equations with 3 Activities and 5 Variations

I wanted to create an activity that I could use to reteach and review angle relationships with some of my students who did not yet show mastery on these concepts.    I decided to create a card set that I can use multiple ways.    Each card has a picture of angles on a point.   They each include adjacent angles, vertical angles, complementary angles, and supplementary angles (with the exception of one card, which does not have complementary angles).   They also each include unknown angle measures.    This allows me to use the cards for five different purposes:  identifying adjacent angles, identifying vertical angles, identifying complementary angles, identifying supplementary angles, and using angle relationships to solve equations.

angle-relationship-cards  .

I can use the activity multiple ways for each concept.

Activity 1 – Give One, Get One 

Students form two lines.   Each student has a card.  I direct them to do a task (identify adjacent angles, identify vertical angles, identify complementary angles, identify supplementary angles, or solve for the unknown angle value)   Each student completes the task on his or her card and then steps forward.   When both partners have stepped forward, they discuss their respective cards/solutions.   When they are done, they step back into the line.    When all pairs have returned to their starting position, one of the two lines shifts (down or up) by one so that they have a new partner.   The cards are then shifted so that they also have a new problem.   This repeats until the allotted time for the activity is complete (usually about 5 minutes).

For this task, I have students use dry erase markers to identify the angles.

Variation 1 – Find complementary angles

Variation 2 – Find supplementary angles

Variation 3 – Find adjacent angles

Variation 4- Find vertical angles

Variation 5 – Find the missing angle measures

Activity 2 – Quiz, Quiz, Trade

Students solve their card.    They then find a partner.   They ask their partner to solve the card (find the angle pair or solve for the missing angle measure).   If the partner has difficulty, they may give a tip (hint).   If the partner still has difficulty, they may give another tip (hint).   If the partner still needs help, they show the partner how to do the problem.   (Tip-tip-tell).   The second member of the pair then quizzes the first partner with his or her card.   After the pair is done, they find new partners.

Variation 1 – Find complementary angles

Variation 2 – Find supplementary angles

Variation 3 – Find adjacent angles

Variation 4- Find vertical angles

Variation 5 – Find the missing angle measures

Activity 3 – War

Students each turn over a card.   They find the missing angle measure.   This requires them to use angle relationships to write an equation to find the missing angle measure.   They then solve the equation and use the value of the variable to find the missing angle measure.   The student who has the card with the greater angle measure wins the cards in that round.   The student with the most cards when I call time is the winner of the game.

For this card set, I used images from an EngageNY lesson to create the cards.

Save

Exploring Misconceptions about Area Part Two: How Does the Surface Area of Cylinder Change When you Double the Height

What happens to the surface area of a cylinder when you double the height?   I posed this question to my students as a Commit and Toss formative assessment last week because I wanted to see if they would realize that while the volume has doubled, the surface area has not.

sa-cylinder-with-double-height-commit-toss

As we discussed the question (which is the final stage of a Commit and Toss formative assessment), I was really pleased that the response I got universally was that the volume doubled but the surface area did not.    They talked about the fact that the area of the bases had not doubled, only the area of the “side” had.    (This was a definite win after the previous Commit and Toss activity – see my last post).

Since things went well, I followed up with the question of how things would change if the radius was doubled.   No one was quite sure, so we took some time to explore the question.   It was a good day.   It’s always a good day when students want to know more.

Exploring Misconceptions: How Does a Change in Dimension Change the Area? Part One

How does the area of a square change if the side length is doubled?     I intended this assessment to draw out common misconceptions.   I expected students to either see it as doubling (disregarding the fact that the side length doubled in two dimensions) or to see it as quadrupled.   I also realized that some students would be stopped in their tracks by the fact that the dimension was a variable and decide there wasn’t enough information.  Hence, all three of those were among the choices on this multiple choice item.

area-of-square-with-double-side-length-commit-toss

Most of the responses that I got were much as expected.   A few students were confused by the variable, a few students said that the area would double and most of them realized that it would quadruple.

As a follow-up question, I asked them to find the area of each square.   I expected this to be unnecessary, really, since they had largely determined that the area would quadruple.   I was really stunned by their responses:   “Four x”,   “Two x squared”,  “Four x squared”.     I was only a little surprised by the “four x”.   My students have experience with exponents but the majority of that experience is with numbers and not variables.   While we have done a little work with raising variables to powers, it is still a little bit novel for some of them so I should have anticipated this error.   However, how could they say that the area quadrupled and have a coefficient of 2?   I’ve spent a fair amount of time considering exactly where the error occurred here.   Was it carelessness and they just forgot about the 2 and then did not pause to consider whether the answer was reasonable?   Was it that they are not making the connection between the quadrupling and the coefficient?   Based on the debrief, I think it was the former but I still find myself wondering if that was all there was to it.   Hence, there will definitely be some sort of follow-up assessment to see if they have really “got it”.

My biggest take-away was a reminder to never assume too much.

When administering the assessment, I used it as a Commit and Toss assessment.   Students write their solution, crumple it in a ball and throw it around the room.  They keep throwing the balls until I tell them to stop so that no one knows whose assessment is whose. Students  examine the work on the assessment they have when time is called.   Then the class debriefs the assessment.

Save

Complementary and Supplementary Angles Foldable

As the second part of my lesson on angles, I had my students complete an advance organizer/foldabel on complementary angles, and supplementary angles.   This is a follow-up to the introduction of the idea of adjacent and vertical angles.   As I said in the previous post, I decided to use this as an opportunity to also review solving two-step equations (something they learned last year but that may have suffered from the summer slide a little bit).   By incorporating ratios in the description of the angle relationship and unknown angle sides, I can embed a little additional practice with ratios, writing equations, and solving equations.

I considered putting all four concepts into a single foldable, but felt like I was pushing the bounds of how much information would fit in a single foldable.    I’m not sure how this is going to go, so I may end up re-doing the whole thing for next year.    Since this is the second of two related foldables, I embedded some of the ideas from the adjacent angle/vertical angle foldable into this one as well.   I wanted to show students that these concepts are all related.

You can download the Complementary and Supplementary Angles foldable by clicking on the text.

 

Adjacent and Vertical Angles Foldable

On Tuesday, I taught my 7th graders about adjacent angles, vertical angles, complementary angles, and supplementary angles.   I decided to use this as an opportunity to also review solving two-step equations (something they learned last year but that may have suffered from the summer slide a little bit).   By incorporating ratios in the description of the angle relationship and unknown angle sides, I can embed a little additional practice with ratios, writing equations, and solving equations.

I created an advance organizer/foldable to use as part of the lesson.

 

You can download the Adjacent and Vertical Angle Foldable by clicking on the text.

I like the structure of the inside of the organizer but am not crazy about the front.   I tried to have kids incorporate color as they completed it.   It was a valiant attempt I guess, but I think they got better as the week wore on.   Today, they did a really nice job using color to show various angle relationships on a point.   I’m not sure if it is that they got better at it or if it is that I got better at it.    This is my first time with this particular course, so I am finding that there are a lot of things I can do better next time.

Tetris Jenga – Reviewing Nets, Surface Area and Volume

I make part of the review for the test on Surface Area and Volume a series of stations that review different concepts from the unit.   One of the stations is a Tetris Jenga game.     This is a variation on the traditional Jenga game in which the blocks are the shape of Tetris blocks.     The variation in shape lends additional challenge to the usual Jenga game.   It also provides some interesting 3-dimensional shapes to explore.

At this station, I have set up the Tetris Jenga tower.   A player draws a block from the tower and must perform the task on the block.   Each block has a label directing the student to make a net, find the surface area of the block, or find the volume of the block.    Because the blocks are 1/2 unit thick, the task also requires students to practice these tasks with fractional values.     I have an answer key at the station so that students can verify the accuracy of their work.

In a normal game of Jenga, successfully drawing out the block ends a turn.   I require the students to correctly find the net, surface area, or volume to keep the block.   I have an answer key with the station so that students can check their result.   If the solution is incorrect, the player must put the block back on the top of the tower.

I also change the winning criteria. Normally, the person who knocks the tower down is out of the game.   I don’t want anyone to stop playing  Instead of being out of the game, the player who knocks down the tower must put all of his or her blocks back and rebuild the tower.   The winner of the game is the player who has amassed the most blocks at the end of the game.  The prize is a piece of candy.

I bring this game out again from time to time after the conclusion of the unit to keep the concepts of nets, surface area, and volume fresh.   I have students play it during the last five to ten minutes of class every so often.   This keeps the concepts fresh for those who have mastered it and gives me a chance to do some re-teaching with students who don’t quite have full mastery at the end of the unit.

Gung Hay Fat Choy, Saint Valentine

Gung Hay Fat Choy, Saint Valentine!   East meets west in the southwest.   It’s actually not quite as strange as it may seem.   First though, a little context because math, like life, makes more sense when seen as part of a larger story.

The Prequel 

My class is in the middle of a unit solving problems relating to surface area and volume.   The unit began by exploring the area of two dimensional shapes (parallelograms, triangles, trapezoids, circles, and irregular or complex shapes).   Next, the class began exploring nets and the area of nets.   The lesson just prior to this lesson had students creating nets for a rectangular prism with a square base and finding the area of the nets.   They explored the idea that the same 3D shape can have many nets but that all the nets have the same area.   Some students found the area by treating the net as an irregular shape (decomposing the net into parts and summing the area of the parts).   Other students summed the area of all the faces (while this is also decomposing the net, it is in a more prescribed way). These were Connected Math lessons (a research-based constructivist curriculum).

The next lesson was slated to be further exploration of rectangular prisms with rectangular (rather than square) bases.   I felt my students were ready for greater challenge but wanted to stick with the plan of allowing them to construct an understanding of surface area.

Gung Hay Fat Choy, Saint Valentine

After a quick entry card in which students had to find the net of a triangular prism, the class watched a short video clip of a dragon dance.   (While they were watching, I touched base with any students who had made an error on the entry card.) Then, we had a brief discussion about the dragon dance and the fact that lunar new year is next week.   We talked a little bit about how different families celebrate the new year.   At that point, I explained that, since next week was both the lunar new year and Valentine’s Day, I was thinking about sending a care package to my daughters away at school.   The care package provided the context for the lesson.   I told them I was thinking of sending a gift card (in lieu of the red envelop filled with money) in a gift card box, a canister of green tea (because oranges in the mail might not be the best idea), a Toblerone (because of course they would expect some chocolate for Valentine’s Day), and a can of green chile (because that is the food they miss most from home).   Since it was a surprise package, it would be fun to wrap everything.   I wanted to keep costs down, though, so I needed to know how much it would cost to wrap each item before making a final decision about the gift wrap.

I created four stations for students.   Each station contained one of the items to go in the care package.   Students needed to create a net, label the faces, and include the dimensions.   Next, they needed to find the area of the net.   Finally, they had to find the cost of the paper to wrap the paper given that the paper cost 1/10 of a cent per square centimeter.   Students in each group had to reach a consensus on the area and cost before moving to the next station.

• The gift card box station was a slight step up in demand from the previous day only in that they had to determine the cost of the paper.   The challenge here was the idea of 1/10 of a cent.   My key questions for this station were:
  • What does the paper cost?
  • What is 1/10 of a cent?
  • What is a reasonable answer?

I expected the most likely error to be students multiplying the area by 1/10 rather than by  1/1000 to find the cost.   That held true.   A shocked expression at the proposal that I pay $23 to wrap something was followed by questions about what it means to be 1/10 of a cent.

 

• The Toblerone station was a slightly bigger step but still very accessible. While this was the first time students would have to find the area of a triangular prism, they had plenty of experience with the creation of different kinds of nets and had figured out that the surface area of a rectangular prism was the area of its net.   I saw this as a relatively small step in thought that they should be able to figure out without any real guidance.   My key questions for this station were:
  • What do you need to measure on the triangular base?
  • Is it enough to measure a single side?   Why is that sufficient or why isn’t it?
  • How can you tell if the triangle is equilateral?
  • Would it impact the net if the sides of the triangle were not congruent?   How?

I expected students to take the easy way out and simply assume that the triangular prism was built on an equilateral triangle base. They pretty much held true to my expectations, so we had some interesting conversations about the need to verify assumptions.

• The green chile and green tea stations were going to be a bigger step.   The larger step was part of my decision to have two stations that were so similar.   I expected the net to be a little bit of a challenge.   I expected some struggle with determining what the “side” of the cylinder would look like in two-dimensional space.   I also expected some struggle with determining the dimensions of the rectangle.   My key questions were:
  • What does the net look like?
  • What are the dimensions of the rectangle?
  • How could you create a model that might help you figure out the dimensions?
  • What dimension should you use for the circle?

Some students readily made the connection to a can label as they grappled with the net and dimensions.   Others played around with the idea of modeling the can.   Most grabbed a piece of paper (often, one of the task cards) to create the model.   Almost all of them then made the connection to the rectangular shape and the idea of the circumference as one of the dimensions.

I thought that we should be able to move through all the stations in a single day, but things took a little longer than expected.   I wanted kids to construct their own understanding at this stage, so I chose to let them take the time necessary to do that.   I extended the lesson into a second day to finish the stations.

I added a concluding activity on the second day summarizing out experience with surface area.   Students completed a foldable comparing and contrasting surface area of rectangular prisms, triangular prisms, and cylinders.

Comparing & Contrasting Surface Area of Prisms and Cylinders

I also created a modified version of the foldable for two of my students.   The modified version provides a little more explicit guidance with regard to some of the sequential steps in finding the area.

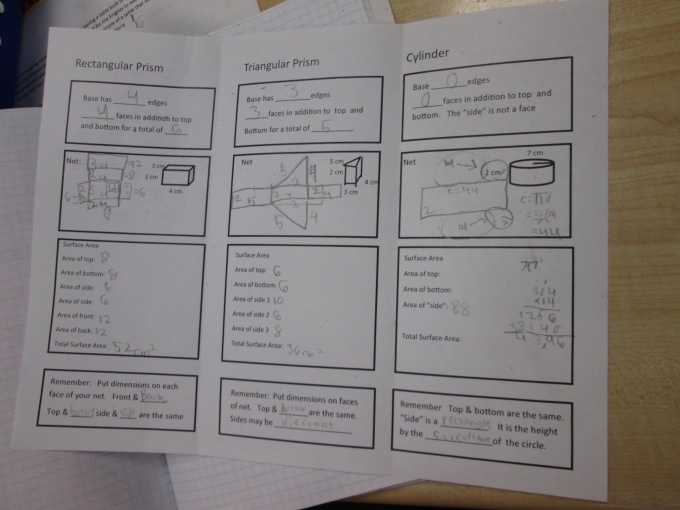
Comparing & Contrasting Surface Area of Prisms and Cylinders Modified

Coming Attractions

Tomorrow, we will explore surface area for a fixed volume.